|
|
|
| Dienstag, 29. Januar 2013 | |
|
|
|
Ernst Eduard Kummer
|
|
|
|
|
|
|

|
|

|
|

|
|
|
|
|

|
|
|
|
|
|
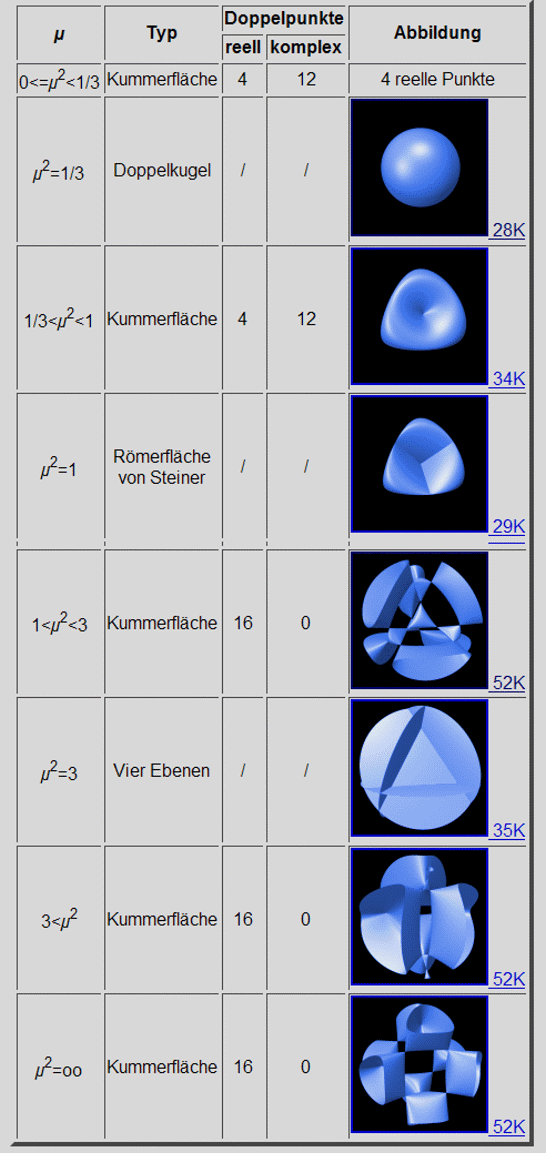
Kummerflächen Die reell-eindimensionale Familie von Flächen vom Grad vier (Quartiken) |
|

|
|
| wurde 1864 von Ernst Eduard Kummer angegeben. Fast jede dieser Flächen besitzt µ(4)=16 gewöhnliche Doppelpunkte, die jedoch im Komplexen liegen können. Variiert man µ, so ergibt sich folgende Tabelle: | |
|
|
|
ABCD Der Beweis von
Kummer ist noch einleuchtender als der von Euklid und so schön kurz und einfach, so dass er unter diesem Gesichtspunkt der beste ist. |
|
|
|
|
|
Despite being called the father of modern arithmetic (that is, number theory), Kummer was rather poor at simple arithmetic. Once, in a class, he needed to find the product of seven and nine. “Seven times nine,” he began, “Seven times nine is er – ah --- ah --- seven times nine is ….” “Sixty-one,” a student suggested.
Kummer wrote 61 on the
board. “Sir,” said another student, “it should be
sixty-nine.”
Paul
Erdős |
|
| Email:
|
|







